
Solve Xcosx Dy Dx Y Xsinx Cosx 1 Youtube
Click here 👆 to get an answer to your question ️ variation of parameter (D^21)y=xsinx sravanasandhya8055 sravanasandhya8055 Math Secondary School answered Variation of parameter (D^21)y=xsinx 1 See answer Advertisement Advertisement Best answer Symbolic form of the given equation, (D2 1) y = xsinx Auxiliary equation, D2 1 = 0 ie D = ±i Thus CF = c1y1 c2y2 = c1cosx c2sinx (1) Let PI = u1(x)y1 u2(x)y2;
(d2-1)y=xsinx(1+x^2)e^x
(d2-1)y=xsinx(1+x^2)e^x-Dividing by y undoes the multiplication by y D^ {2}=\frac {\left (x^ {2}1\right)e^ {x}x\sin (x)y} {y} D 2 = y ( x 2 1) e x x s i n ( x) y Take the square root of both sides of the equation Take the square root of both sides of the equationSee the answer See the answer See the answer done loading
2
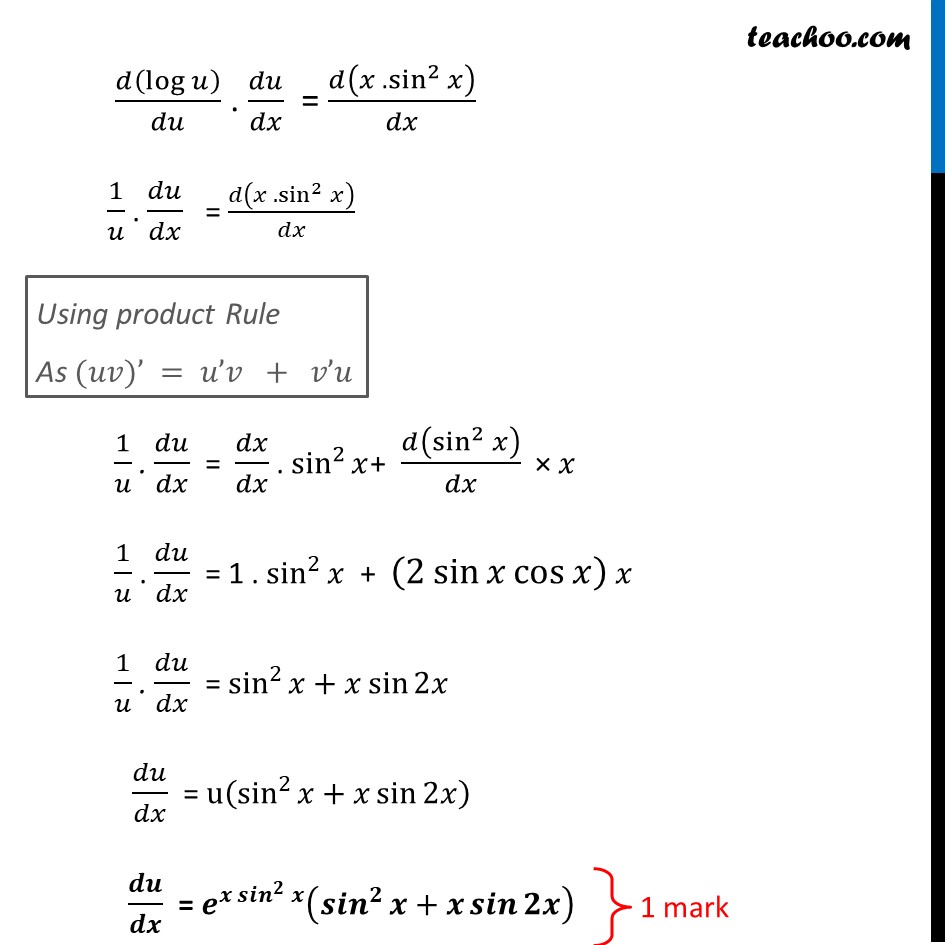
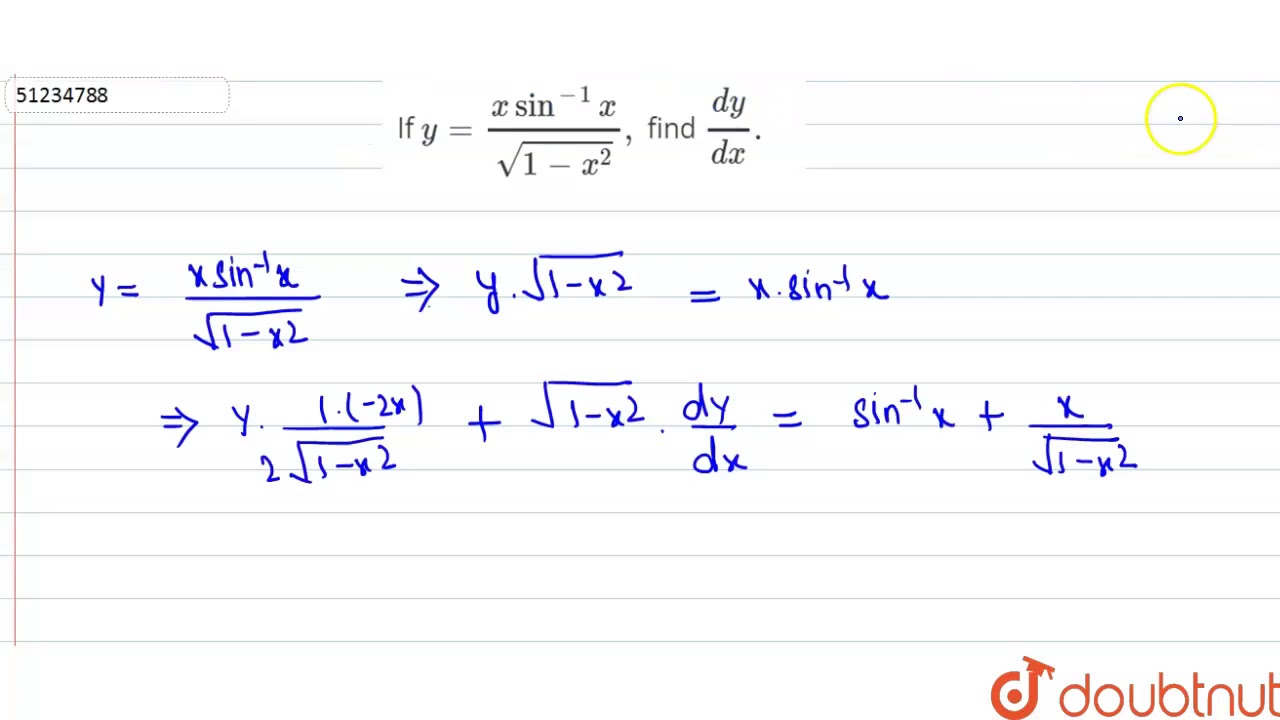
Complimentary Function The homogeneous equation associated with A is d2y dx2 2 dy dx 5y = 0 And it's associated Auxiliary equation is m2 2m 5 = 0 Which has two complex solutions m = −1 ± 2i Thus the solution of the homogeneous equation is yc = e−1x(Acos2x Bsin2x) = Ae−x cos2x Be−xsin2xY = d d x ( sin x × log e x) In this differentiation problem, the variable y represents a function in x Hence, it can be differentiated with respect to x and do not think that y is a constant Therefore, the function y can be differentiated by the derivative rule of logarithms 1 y × d y d x = d d x ( sin Find a particular solution to the equation $$(D^22D1)y=x\sin x$$ \begin{align} \text{PI}&=\frac{1}{(D^22D1)}x\sin x\\ &=\frac{1}{(D1)^2}x\sin x\\ &=\text{IP
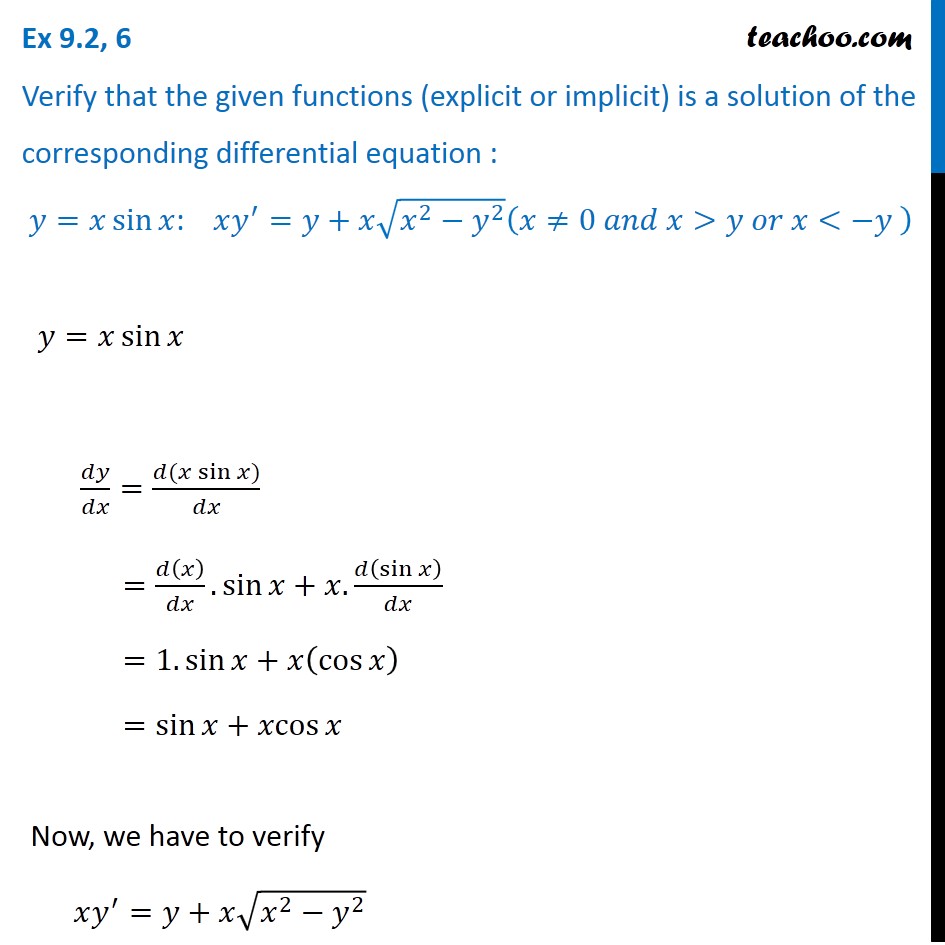
Question Solve the following differential equations a) d^2y/dx^2 y = xe^x sin2x B) d^2y/dx^2 y = e^x (1x^2) xsinx This problem has been solved!Share Report Share Answer this doubt The given differential equation is,The auxiliary equation ism2– 1 = 0orC1e–xC2ex (asC1,C2are arbitrary constant)Hence,y=CFPIgives the You can study other questions, MCQs, videos and tests for Electrical Engineering (EE) on EduRev and even discuss your questions like (D^21)y=xsinx (1 x^2)e^x?
(d2-1)y=xsinx(1+x^2)e^xのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 | ||
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | 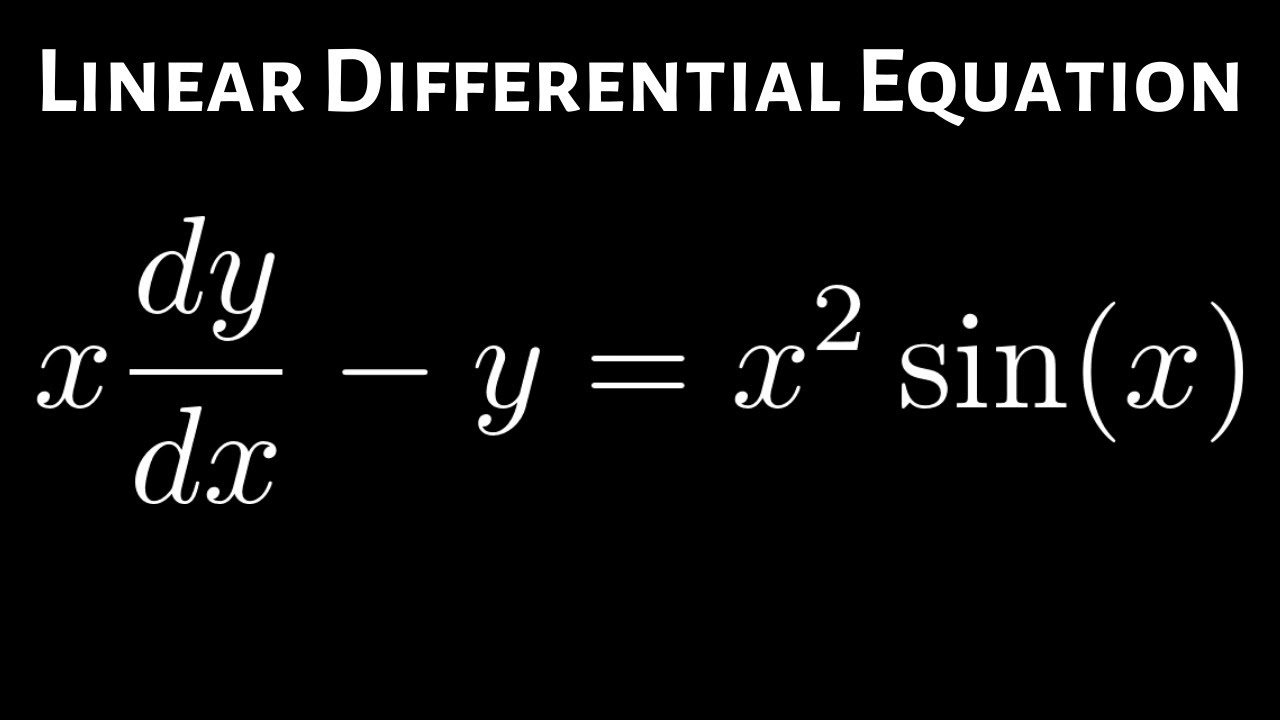 |
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 | ||
 | ||
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 |  |  |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | 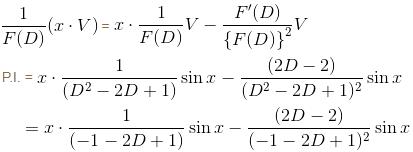 |
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
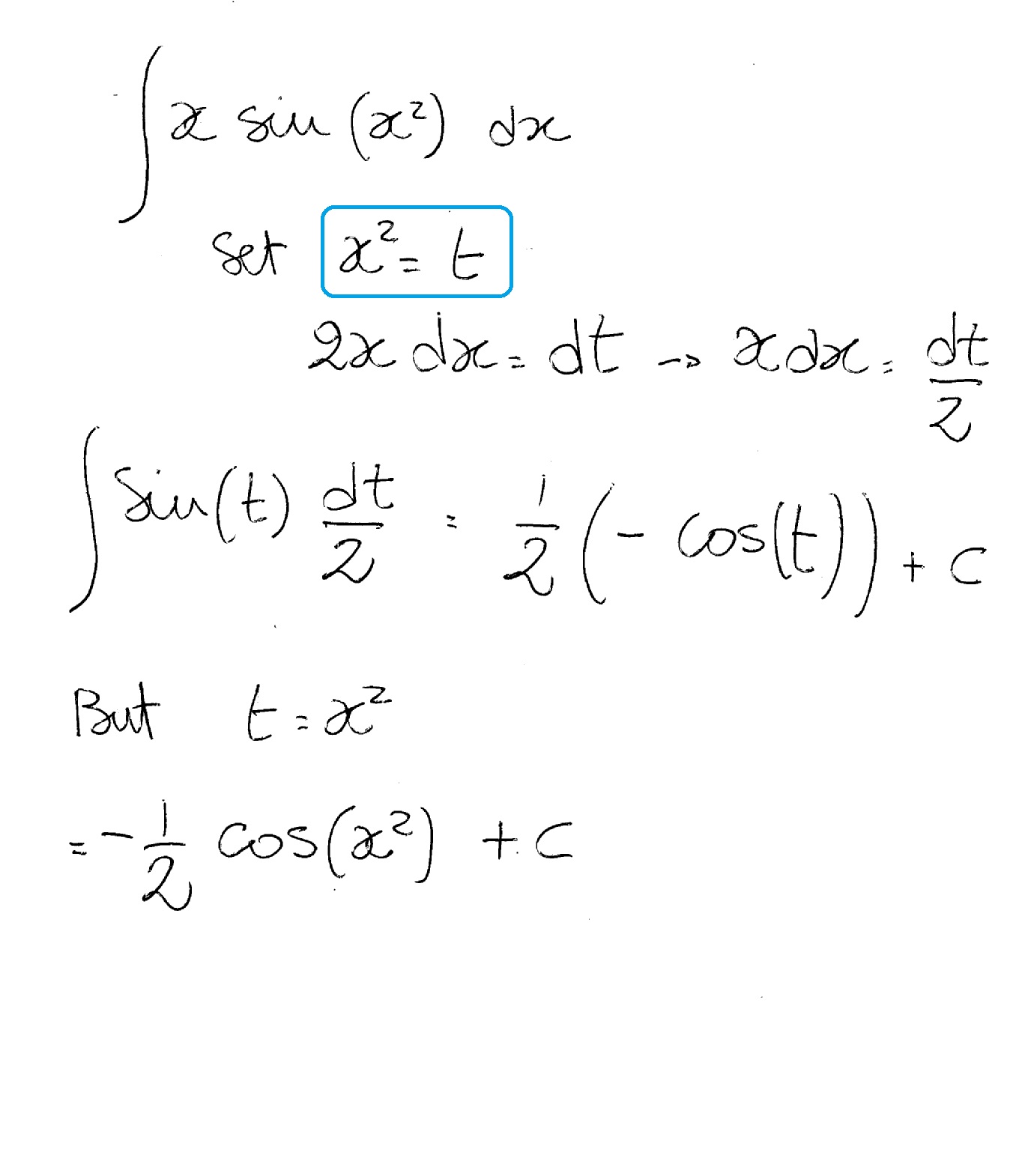 | ||
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 |  | |
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  | |
 | ||
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 | ||
 | ||
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
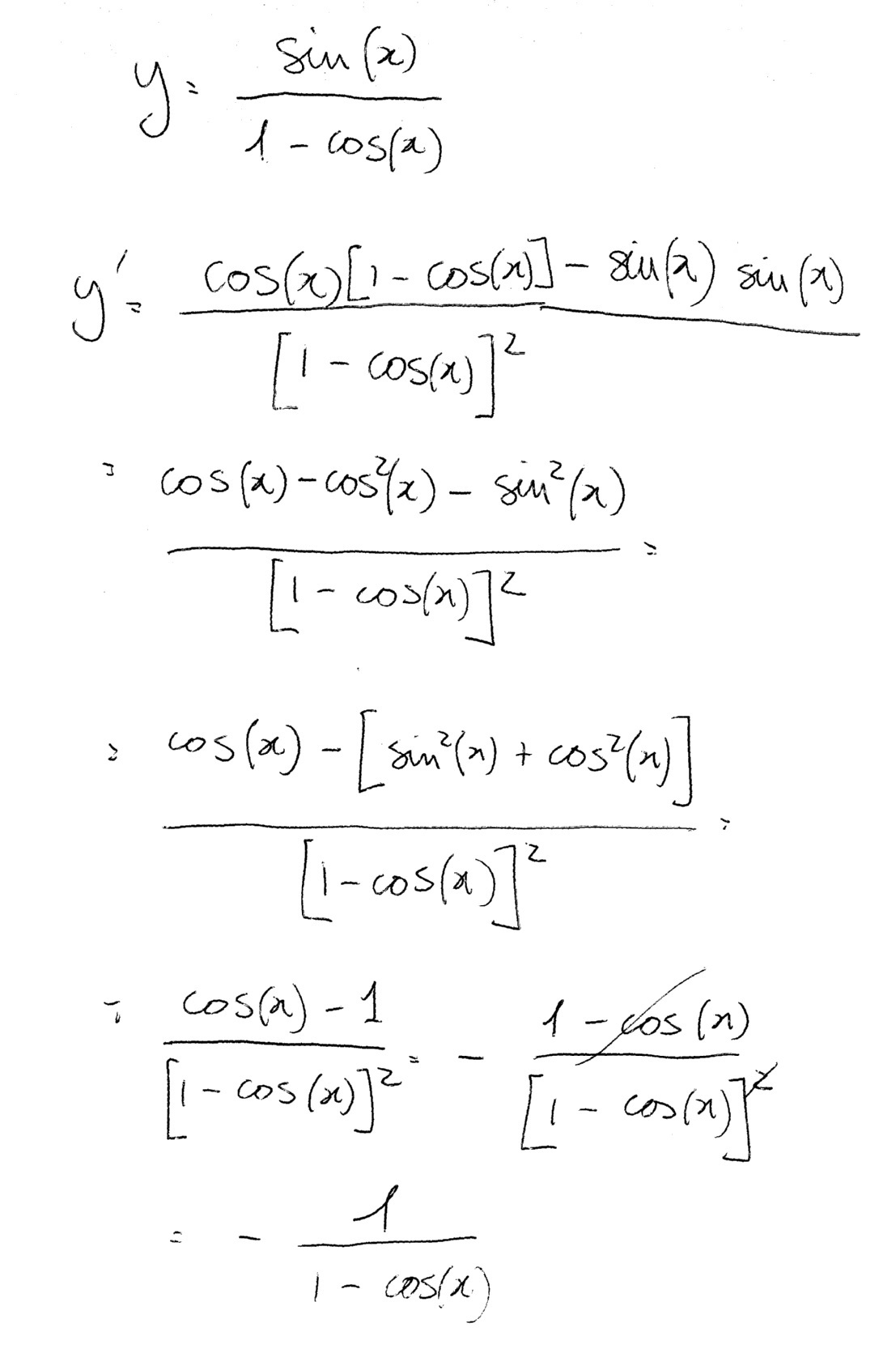 |  | |
 | ||
「(d2-1)y=xsinx(1+x^2)e^x」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |
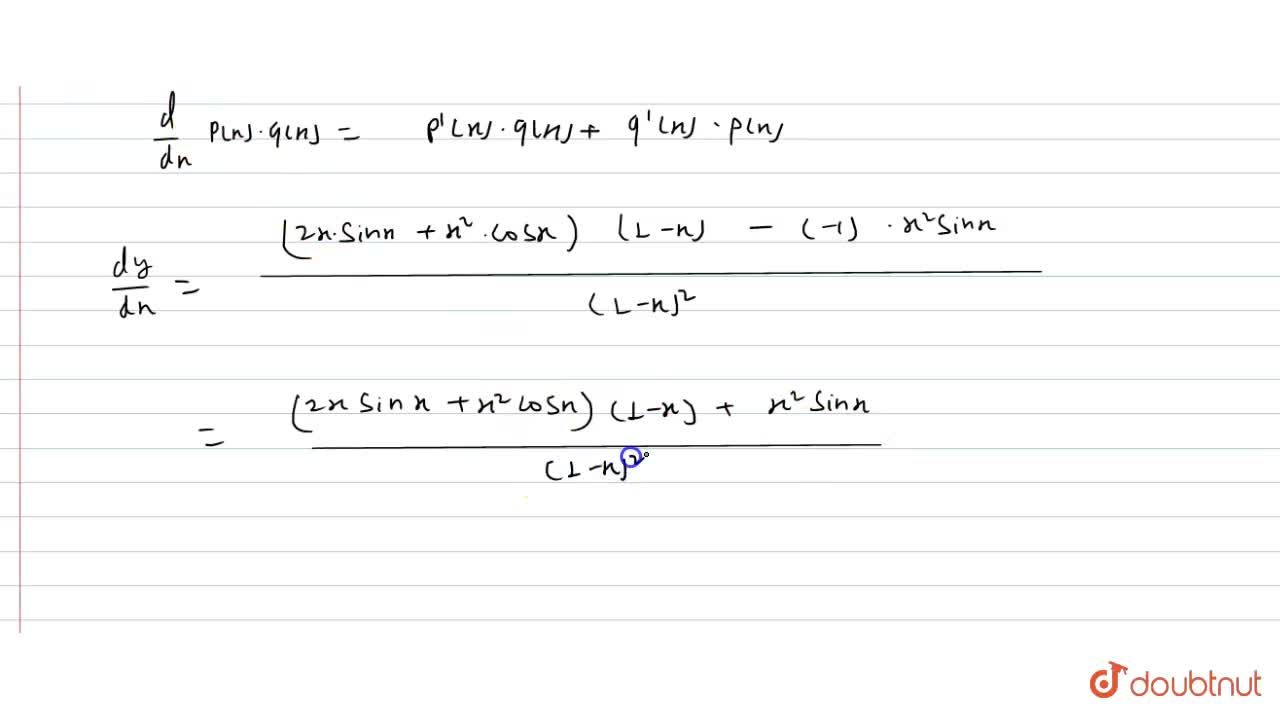
Y" 2y' 2y = 0 auxiliary equ is> D^2 2D 2 =0 D = {2 (4 8)^1/2 } /2 D = {2 2i } /2 D1 = (1i) and D2 = (1 i) you are NOT right, the general solution is> y = c1 e^(1i)x c2 e^(1i)x y = e^xc1 e^ix c2 e^ix View the full answer" The first times the derivative of the second plus the derivative of the first times the second " So with y = xsinx ;
Incoming Term: (d2-1)y=xsinx(1+x^2)e^x, (d^2-1)y=xsinx+x^2e^x,




No comments:
Post a Comment